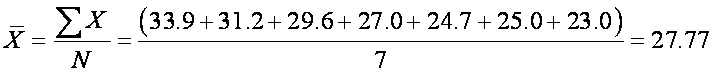PEP 6305 Measurement in
Health & Physical Education
Topic 3:
Percentiles & Measures of Central Tendency
Section
3.2
Click to go to
back to the previous section (Section 3.1)
n
Central tendency
is a way to describe the
midpoint of a distribution.
n
Measures of central tendency are used to describe performance or
ability for a group of subjects rather than describing any individual subject
or the whole range of values.
n
Three measures of central tendency:
¨
Mode: the most frequently observed score. To
identify the mode, look at the simple frequency distribution and find the value
that occurs most
frequently. Works best for
nominal-scale data.
¨
Median: the 50th
percentile; the score at which half the scores lie above and half below.
To identify the median, find the
midpoint of the rank order distribution. Works best for ordinal-scale data, or interval-scale or ratio-scale
data that is highly
skewed.
¨
Mean: the average score. Works best for
continuous, symmetrically distributed,
interval-scale or
ratio-scale data. Many
variables in health and kinesiology have these characteristics, so the mean is
the most frequently reported measure of central tendency.
n
The symbol for the mean is a bar over the variable symbol (in this
case, X): 
.
The mean is calculated by summing all of the scores ( ∑ X ) and dividing by the
total number of scores (N):
n
So, for the example given on page 55 of the text:
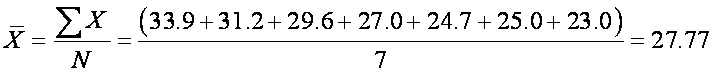
n
Using the formula, calculate the mean of the data for which you
computed the percentiles above. (Answer)
Relations Among the
Measures of Central Tendency
n
The mode, median, and mean are all approximately the same value
for interval or ratio scale data that are normally (or even just symmetrically)
distributed.
n
When the data distribution is
skewed, these values are not the
same.
¨
For positively skewed data, the mean has a higher value
than the median, and the median has a higher value than the mode.
¨
For negatively skewed data, the mean has a lower value than the
median, and the median has a lower value than the mode.
Positive skew: Mode < Median < Mean
Negative skew: Mean < Median < Mode
n
How do you know which measure of central tendency to use?
¨
For continuous data that are symmetrically distributed, use the
mean.
¨
For ordinal data or continuous data that are highly skewed, the
median
may be a better representation of the group because it is not influenced by
extreme values.
¨
For nominal data, use the mode or, even better, describe the frequency
or proportion of subjects in each
category (i.e. the grouped frequency distribution).
n
The mean and the median (50th percentile) can be produced using
R Commander; they
were printed when you did the deciles example.
¨
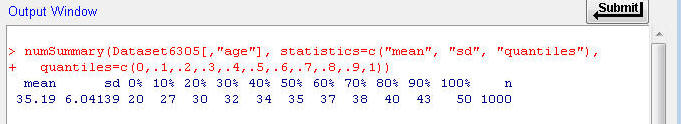
You can see (I hope) that the Mean = 35.19, and
the Median (50%-tile) = 35. You can use Excel to order the data in a column and
then look to identify the most common value if you need to find the Mode (the
most frequent score).
Formative
Evaluation
n
List the advantages and disadvantages of the mode, median, and
mean as measures of central tendency (see text, p. 56-57). Describe the data scale type
appropriate for each.
n
Give an example of a variable for which you would
compute the mode, median, and mean.
n
How do you decide whether to use the mode, median, or mean to
represent central tendency?
You have reached the end of Topic 3.
Make sure to work through the Formative Evaluation
above and the textbook problems (end of the chapters). (remember how to
enter data into R Commander?)
You must complete the review quiz (in the Quizzes
folder on the Blackboard course home page) before you can advance to the next topic.